- Lesson One: The Number One
- ... in Geometry
- ... in Nature
- ... in Culture
- "Uroboros" (related artwork by the author)
- "Creation" (related artwork by the author)
- Lesson Two: The Square Root of Two
- ... in Geometry
- ... in Nature, Part 1
- ... in Nature, Part 2
- ... in Culture, Part 1
- ... in Culture, Part 2
- "Duality" (related artwork by the author)
- Lesson Three: The Square Root of Three
- ... in Geometry
- ... in Nature
- ... in Culture
- "Vesica" (related artwork by the author)
- Lesson Four: Phi, or the Golden Proportion
- ... Intro, Part 2
- ... Intro, Part 3
- ... in Geometry
- ... in Nature
- ... in Nature, Part 2
- ... in Culture
- "The Flower of Phi" (related artwork by the author)
- Lesson Five: The Five Perfect Solids
- ... Intro, Part 2
- ... The Tetrahedron
- ... The Octahedron
- ... The Cube
- ... The Icosahedron
- ... The Dodecahedron
In order to facilitate understanding of the three-dimensional nature of the Platonic Solids, many animations have been included in this section. These animations may take longer to download than the still images on this website. Your patience is appreciated.
With the exception the discussion of the sphere and cube as symbols of unity, there has been very little mention of three-dimensional geometry up until this point in this body of work. All of the transcendent irrational proportions that we have been discussing have been dealt with as they relate to two-dimensional geometric figures. With the five Perfect, or "Platonic" Solids, we take the step of applying the ideals of sacred geometry to three-dimensional figures.
Before we describe the details of each of these five shapes, it is important that we define what it is about them that sets them apart from all other three-dimensional shapes. But before we delve into that, let us talk a little bit about the history of the five solids, and how they came to be called "Platonic."
The term "Platonic," as many people know, refers to the great Greek philosopher Plato. The five perfect solids have been labeled as "Platonic" due to the heavy importance that Plato placed on these shapes. He expounded upon their various virtues at length in his various works (especially so in Timaeus), and is attributed by many to have been the first to assign one of the four traditional elements (fire, air, water, earth) to each of the shapes, with the fifth, all encompassing, element (spirit) being attributed to the remaining solid.
There can be no doubt that Plato did indeed give special attention to these solids in his work. However, it is an unfortunate aspect of Western culture that we tend to attribute many discoveries to a Greek or Roman source, despite the fact that there is evidence that earlier cultures were well aware of the discoveries irresponsibly attributed to them. As an example, it is a fact that approximately 300 examples of the five perfect solids carved from stone have been unearthed in Scotland, whose dates of creation have been set at more than 1000 years earlier than Plato - making these solids far from a "Platonic" discovery. It also seems very likely that Plato learned of the shapes from Pythagoras, who himself was educated in the ways of sacred geometry by the Egyptians, making their true discoverers (if we can use such a term at all) the Egyptians themselves. This should come as no surprise, considering the depth of the ancient Egyptian's geometric understanding, proof of which is blatantly displayed in so many of their artistic and architectural achievements.
However, despite being a misnomer, the name "Platonic" has become the standard to delineate these five shapes from all others, and in order to avoid confusion, we too will refer to these five polyhedra by this name.
To define these the Platonic solids in geometric terms, we can say that they are the only five polyhedra that are completely regular convex polyhedra. The term “regular” refers to the fact that every face, every edge length, every facial angle, and every dihedral angle (angle between two faces) are equal to all the others that constitute the polyhedra. The term “convex” refers to the fact that all of the sides of the shapes are flat planes, i.e., they are not “concave”, or dented in.
To more easily understand these ideas, let's take a look at some basic individual animations of each of the shapes rotating on their central axis.
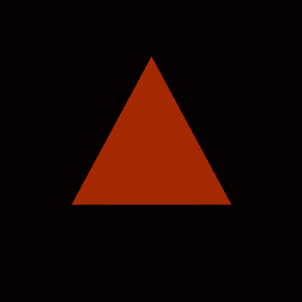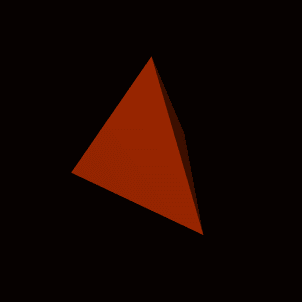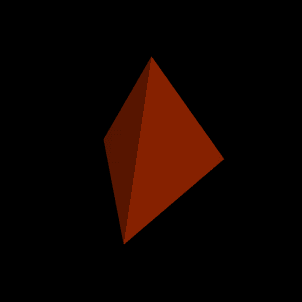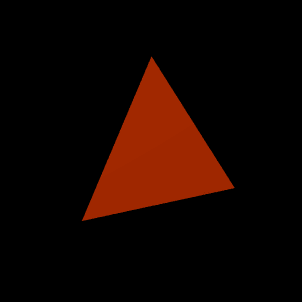 Original animation created by A. O'Connor for Nature's Word using POV-Ray, WWW Gif Animator, and Adobe Photoshop 5.0.
Original animation created by A. O'Connor for Nature's Word using POV-Ray, WWW Gif Animator, and Adobe Photoshop 5.0.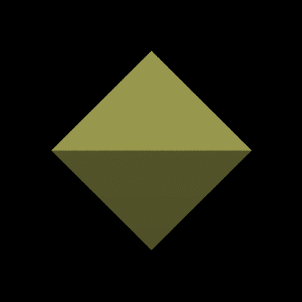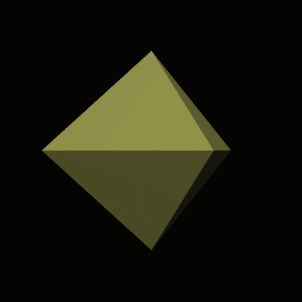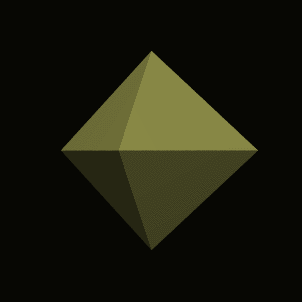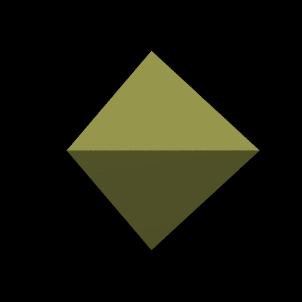 Original animation created by A. O'Connor for Nature's Word using POV-Ray, WWW Gif Animator, and Adobe Photoshop 5.0.
Original animation created by A. O'Connor for Nature's Word using POV-Ray, WWW Gif Animator, and Adobe Photoshop 5.0. Original animation created by A. O'Connor for Nature's Word using POV-Ray, WWW Gif Animator, and Adobe Photoshop 5.0.
Original animation created by A. O'Connor for Nature's Word using POV-Ray, WWW Gif Animator, and Adobe Photoshop 5.0. Original animation created by A. O'Connor for Nature's Word using POV-Ray, WWW Gif Animator, and Adobe Photoshop 5.0.
Original animation created by A. O'Connor for Nature's Word using POV-Ray, WWW Gif Animator, and Adobe Photoshop 5.0. Original animation created by A. O'Connor for Nature's Word using POV-Ray, WWW Gif Animator, and Adobe Photoshop 5.0.
Original animation created by A. O'Connor for Nature's Word using POV-Ray, WWW Gif Animator, and Adobe Photoshop 5.0.To understand why only these specific five shapes can be considered “regular convex polyhedra,” it may be easiest to experiment with making the shapes out of pieces of cardstock. This practice is highly recommended, as it leads to the most thorough understanding of the geometry of the solids. Here are the full patterns, or “nets,” that can be traced on cardstock, cut out, and folded to create each of the five shapes. If an accurate model is to be created, it is best to carefully cut out the patterns and then score the lines to be folded using a ballpoint pen (or similar scoring implement) and a metal straightedge.
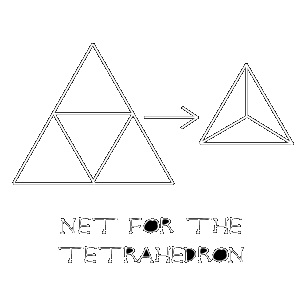 scanned image reworked by A. O'Connor in Photoship 5.0, Taken from: Anthony Pugh's book Polyhedra; A Visual Approach
scanned image reworked by A. O'Connor in Photoship 5.0, Taken from: Anthony Pugh's book Polyhedra; A Visual Approach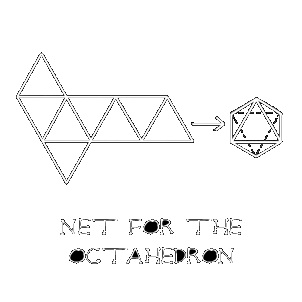 scanned image reworked by A. O'Connor in Photoship 5.0, Taken from: Anthony Pugh's book Polyhedra; A Visual Approach
scanned image reworked by A. O'Connor in Photoship 5.0, Taken from: Anthony Pugh's book Polyhedra; A Visual Approach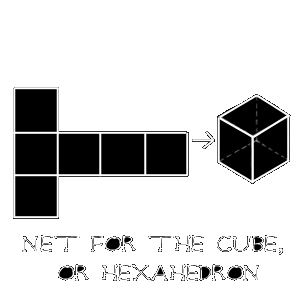 scanned image reworked by A. O'Connor in Photoship 5.0, Taken from: Anthony Pugh's book Polyhedra; A Visual Approach
scanned image reworked by A. O'Connor in Photoship 5.0, Taken from: Anthony Pugh's book Polyhedra; A Visual Approach scanned image reworked by A. O'Connor in Photoship 5.0, Taken from: Anthony Pugh's book Polyhedra; A Visual Approach
scanned image reworked by A. O'Connor in Photoship 5.0, Taken from: Anthony Pugh's book Polyhedra; A Visual Approach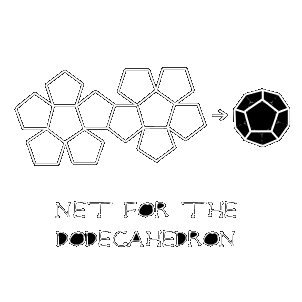 scanned image reworked by A. O'Connor in Photoship 5.0, Taken from: Anthony Pugh's book Polyhedra; A Visual Approach
scanned image reworked by A. O'Connor in Photoship 5.0, Taken from: Anthony Pugh's book Polyhedra; A Visual ApproachOnce we have experimented a bit with these “nets,” it becomes easier to understand why the Platonic solids are the only five regular convex polyhedra. Because every edge of every face must be equal to one another in order for the polyhedron to be fully regular, we are restricted to using only perfect polygons for each face, such as the equilateral triangle, perfect square, and perfect pentagon. Obviously a minimum of three such polygons must touch one another at every vertice (or point) of the solid – two polygons alone would not create a three-dimensional shape. So, if we start with the equilateral triangle, it becomes clear that we can place either three, four, or five triangles around a given point and use this net to create a three-dimensional pyramid-type shape (either a three-, four-, or five-sided pyramid). If we try to place six equilateral triangles around one point, we create a perfect hexagon – a flat plane – once again taking the possibility of three-dimensionality out of the picture. If we look at the animations above, we can see that the tetrahedron groups three triangles around each vertice, the octahedron groups four, and the icosahedron groups five.
Moving on to experiment with the perfect square, we can immediately see that only three squares can border a single point, as four gives us a flat plane and eliminates the possibility of creating a three-dimensional shape. If we look at the animation of the cube above, it can be seen that the cube utilizes three squares gathered around each vertice to create its overall form.
Pentagons also can only be grouped in threes around a given point. To use four creates an overlap, making the creation of a regular three-dimensional solid impossible. The animation of the dodecahedron shows it to be a polyhedron formed of three pentagons arranged around each of its vertices.
If we try to use hexagons gathered around one point, we find that using three such six-sided shapes gives us a flat plane. If we try to use any regular polygons with more than six sides we find that three such polygons cause an overlap in a similar way to using four pentagons.
Thus we have the only five shapes that can have all edge lengths equal, all facial angles equal, all dihedral angles equal, and the same number of faces gathered around every vertice.
Besides the fact that these are the only three-dimensional shapes that can possibly be created that are completely regular in every way, there is much more that makes these specific polyhedra stand out from others. It is the nature of all of the five Platonic solids that each can be used to generate any of the other four solids, either encased within or encasing the original solid. In other words, each solid can be placed within each of the other solids in such a way that its vertices will exactly touch either the center of the surrounding solid’s faces, the midpoints of its edges, or the vertices of the surrounding solid. Another surprising aspect of the solids is the recurrence of the transcendent irrational proportions that we find in their forms.
To understand both of these issues, it is important to be familiar with three possible spheres that are defined in every Platonic solid - the insphere, the intersphere, and the circumsphere. The insphere is defined by the radius that extends from the center point of the solid to the center point of each face, and is completely encased within the solid, with the outer side of the sphere just touching the inside of the center point of each face. In the image, the red dots mark the points where the insphere is touching the inside of the blue icosahedron's faces.
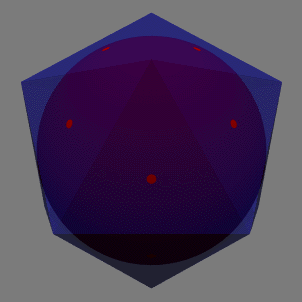 Original artwork created by A. O'Connor for Nature's Word using POV-Ray and Adobe Photoshop 5.0.
Original artwork created by A. O'Connor for Nature's Word using POV-Ray and Adobe Photoshop 5.0.The intersphere is defined by the radius that extends from the center point of the solid to the center point of any edge of the solid.
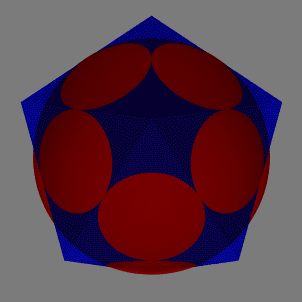 Original artwork created by A. O'Connor for Nature's Word using POV-Ray and Adobe Photoshop 5.0.
Original artwork created by A. O'Connor for Nature's Word using POV-Ray and Adobe Photoshop 5.0.The circumsphere is the sphere that is defined by the radius that extends from the center of the solid to any vertice, and effectively encases the solid, with each vertice just touching the inside of the sphere. In the image, the blue points show where the icosahedron's vertices touch the red circumsphere. Although all three images use the example of the three spheres as they relate to the icosahedron, it is important to note that these spheres can be defined in all of the five Platonic solids.
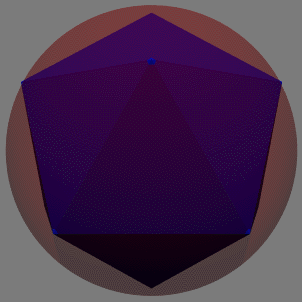 Original artwork created by A. O'Connor for Nature's Word using POV-Ray and Adobe Photoshop 5.0.
Original artwork created by A. O'Connor for Nature's Word using POV-Ray and Adobe Photoshop 5.0.As an example, if we take a dodecahedron with a circumsphere that measures 1, we can place it within an icosahedron whose insphere equals 1, and the points of the dodecahedron will touch the exact center of the each of the icosahedron's faces. To state this another way completely, if we were to connect the center points of all of the icosahedron's faces to those that are adjacent to them, we would define a perfect dodecahedron.
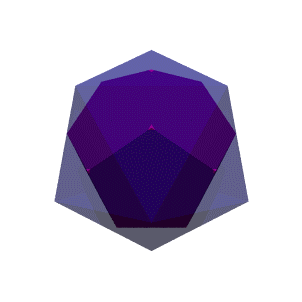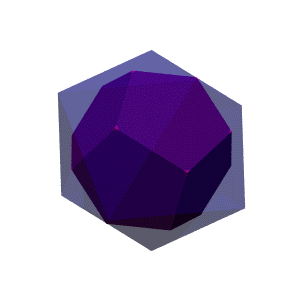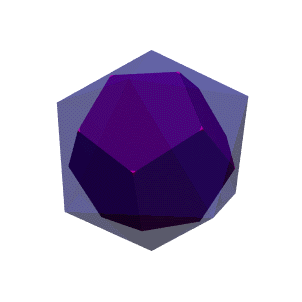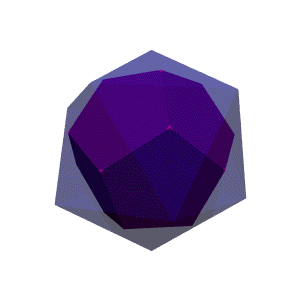 Original animation created by A. O'Connor for Nature's Word using POV-Ray, WWW Gif Animator, and Adobe Photoshop 5.0.
Original animation created by A. O'Connor for Nature's Word using POV-Ray, WWW Gif Animator, and Adobe Photoshop 5.0.These two shapes are not the only Platonic solids that this type of alignment can be observed in - every one of the five shapes can be used to easily define any other of the solids.
 Original animation created by A. O'Connor for Nature's Word using POV-Ray, WWW Gif Animator, and Adobe Photoshop 5.0.
Original animation created by A. O'Connor for Nature's Word using POV-Ray, WWW Gif Animator, and Adobe Photoshop 5.0.The combination of the icosahedron and dodecahedron
 Original animation created by A. O'Connor for Nature's Word using POV-Ray, WWW Gif Animator, and Adobe Photoshop 5.0.
Original animation created by A. O'Connor for Nature's Word using POV-Ray, WWW Gif Animator, and Adobe Photoshop 5.0.The combination of the octahedron and the cube.
 Original animation created by A. O'Connor for Nature's Word using POV-Ray, WWW Gif Animator, and Adobe Photoshop 5.0.
Original animation created by A. O'Connor for Nature's Word using POV-Ray, WWW Gif Animator, and Adobe Photoshop 5.0.The combination of two opposing tetrahedrons.
Another related aspect to the solids is that they form geometric "duals" of one another. If a cube and an octagon with equal interspheres share the same center point, each of their vertices will mark the exact center point of the opposing shape. The same goes for the icosahedron and dodecahedron. When it comes to the tetrahedron, however, we have a slightly different situation - because the tetrahedron is its own dual. If we take two tetrahedrons and rotate one so that it points straight down and leave the other pointing straight up, and then we place them together to share the same center point, the two shapes act as geometric duals for one another. This arrangement makes a sort of three-dimensional "Star of David," and is sometimes referred to as a "star tetrahedron."
If we take apart all of the obvious proportions in the solids, we find that the transcendent irrationals come up over and over again. A related characteristic of the solids is that golden rectangles can be inserted into some of them in certain configurations so that they define the various vertices and edges of the shape. However, rather than complicate this general introduction with complex mathematics and diagrams, we will save the details of how this occurs for the sections dedicated to each individual solid.


